5.7.1 Unit Hyperbola Definitions 🔧
Hyperbolic trig functions operate similarly as the trig functions on the unit circle, except they neither rotate nor have periodicity.

$$\sinh(\theta)=y/h\qquad\text{csch}(\theta)=h/y$$
$$\cosh(\theta)=x/h\qquad\text{sech}(\theta)=h/x$$
$$\tanh(\theta)=y/x\qquad\coth(\theta)=x/y$$
$$\theta=\sinh^{-1}(y/h)\qquad\theta=\text{csch}^{-1}(h/y)$$
$$\theta=\cosh^{-1}(x/h)\qquad\theta=\text{sech}^{-1}(h/x)$$
$$\theta=\tanh^{-1}(y/x)\qquad\theta=\coth^{-1}(x/y)$$
Relations
A unit circle rotated one half radian on the unit double cone along its tangential axis results in the unit hyperbola.

The number $1$ rotated one half radian in the complex plane results the imaginary unit $i$.

The insertion of $i$ into the unit circle equation results in the unit hyperbola equation.
$$x^2+(i\cdot y)^2=1\medspace\to\medspace x^2-y^2=1$$
5.7.2 Hyperbolic Common Identities 🔧
Right Angle Identities
$$\sinh^2(\theta)=\cosh^2(\theta)+1$$
$$\tanh^2(\theta)+\text{sech}^2(\theta)=1$$
$$\coth^2(\theta)=\text{csch}^2(\theta)+1$$
Sum & Difference Identities
$$\sinh(a\pm b)=\sinh(a)\cdot\cosh(b)\pm\cosh(a)\cdot\sinh(b)$$
$$\cosh(a\pm b)=\cosh(a)\cdot\cosh(b)\pm\sinh(a)\cdot\sinh(b)$$
5.7.3 Limits of Hyperbolic Functions 🔧
$$\lim\limits_{\theta\to 0}\frac{\sinh(\theta)}{\theta}=1\qquad\lim\limits_{\theta\to 0}\frac{\cosh(\theta)-1}{\theta}=0$$
5.7.4 Derivatives of Hyperbolic Functions 🔧
$$\frac{d}{dx}\sinh(\theta)=\cosh(\theta)$$
$$\frac{d}{dx}\cosh(\theta)=\sinh(\theta)$$
$$\frac{d}{dx}\text{sech}(\theta)=-\text{sech}(\theta)\cdot\tanh(\theta)$$
$$\frac{d}{dx}\text{csch}(\theta)=-\text{csch}(\theta)\cdot\coth(\theta)$$
$$\frac{d}{dx}\tanh(\theta)=\text{sech}^2(\theta)$$
$$\frac{d}{dx}\coth(\theta)=-\text{csch}^2(\theta)$$
Proof of Sine
Insert $\sinh(x)$ into the derivative function
$$\lim\limits_{\Delta x\to 0}\frac{\sinh(x+\Delta x)-\sinh(x)}{\Delta x}$$
Substitute the sum identity for $\sinh(x)$
$$\lim\limits_{\Delta x\to 0}\frac{\sinh(\Delta x)\cdot\cosh(x)+\sinh(x)\cdot\cosh(\Delta x)-\sinh(x)}{\Delta x}$$
Factor into terms of hyperbolic trig limits
$$\lim\limits_{\Delta x\to 0}\bigg(\frac{\sinh(\Delta x)}{\Delta x}\cdot\cosh(x)+\frac{\cosh(\Delta x)-1}{\Delta x}\cdot\sinh(x)\bigg)$$
Solve the limits
$$1\cdot\cosh(x)+0\cdot\sinh(x)$$
Simplify
Proof of Cosine
Insert $\cosh(x)$ into the derivative function
$$\lim\limits_{\Delta x\to 0}\frac{\cosh(x+\Delta x)-\cosh(x)}{\Delta x}$$
Substitute the sum identity for $\cosh(x)$
$$\lim\limits_{\Delta x\to 0}\frac{\cosh(\Delta x)\cdot\cosh(x)+\sinh(\Delta x)\cdot\sinh(x)-\cosh(x)}{\Delta x}$$
Factor into terms of hyperbolic trig limits
$$\lim\limits_{\Delta x\to 0}\bigg(\frac{\cosh(\Delta x)-1}{\Delta x}\cdot\cosh(x)+\frac{\sinh(\Delta x)}{\Delta x}\cdot\sinh(x)\bigg)$$
Solve the limits
$$0\cdot\cosh(x)+1\cdot\sinh(x)$$
Simplify
Proof of Secant
Proof of Cosecant
Proof of Tangent
Proof of Cotangent
5.7.5 Real Version of Euler's Formula 🔧
$$e^\theta=\cosh(\theta)+\sinh(\theta)$$
Proof
5.7.6 Analytic Hyperbolic Functions 🔧
$$\sinh(\theta)=-i\cdot\sin(i\cdot\theta)=\frac{e^\theta-e^{-\theta}}{2}$$
$$\cosh(\theta)=\cos(i\cdot\theta)=\frac{e^\theta+e^{-\theta}}{2}$$
$$\text{sech}(\theta)=\sec(i\cdot\theta)=\frac{2}{e^\theta+e^{-\theta}}$$
$$\text{csch}(\theta)=i\cdot\csc(i\cdot\theta)=\frac{2}{e^\theta-e^{-\theta}}$$
$$\tanh(\theta)=-i\cdot\tan(i\cdot\theta)=\frac{e^\theta-e^{-\theta}}{e^\theta+e^{-\theta}}$$
$$\coth(\theta)=i\cdot\cot(i\cdot\theta)=\frac{e^\theta+e^{-\theta}}{e^\theta-e^{-\theta}}$$
Proof of Sine and Cosine
Proof of Secant, Cosecant, Tangent, and Cotangent
5.7.7 Analytic Hyperbolic Inverses 🔧
$$\sinh^{-1}(\theta)=\ln\big(\theta+\sqrt{\theta^2+1}\big),\medspace\forall\theta$$
$$\cosh^{-1}(\theta)=\ln\big(\theta+\sqrt{\theta^2-1}\big),\medspace\theta\ge1$$
$$\text{sech}^{-1}(\theta)=\ln\big(\theta^{-1}+\sqrt{\theta^{-2}-1}\big),\medspace 0\lt\theta\lt1$$
$$\text{csch}^{-1}(\theta)=\ln\big(\theta^{-1}+\sqrt{\theta^{-2}+1}\big),\medspace\theta\ne0$$
$$\tanh^{-1}(\theta)=\frac{1}{2}\cdot\frac{1+\theta}{1-\theta},\medspace|\theta|\lt1$$
$$\coth^{-1}(\theta)=\frac{1}{2}\cdot\frac{\theta+1}{\theta-1},\medspace|\theta|\gt1$$
Proof of Sine
Proof of Cosine
Proof of Secant
Proof of Cosecant
Proof of Tangent
Proof of Cotangent

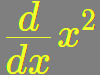





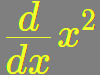



 $$\sinh(\theta)=y/h\qquad\text{csch}(\theta)=h/y$$
$$\cosh(\theta)=x/h\qquad\text{sech}(\theta)=h/x$$
$$\tanh(\theta)=y/x\qquad\coth(\theta)=x/y$$
$$\theta=\sinh^{-1}(y/h)\qquad\theta=\text{csch}^{-1}(h/y)$$
$$\theta=\cosh^{-1}(x/h)\qquad\theta=\text{sech}^{-1}(h/x)$$
$$\theta=\tanh^{-1}(y/x)\qquad\theta=\coth^{-1}(x/y)$$
$$\sinh(\theta)=y/h\qquad\text{csch}(\theta)=h/y$$
$$\cosh(\theta)=x/h\qquad\text{sech}(\theta)=h/x$$
$$\tanh(\theta)=y/x\qquad\coth(\theta)=x/y$$
$$\theta=\sinh^{-1}(y/h)\qquad\theta=\text{csch}^{-1}(h/y)$$
$$\theta=\cosh^{-1}(x/h)\qquad\theta=\text{sech}^{-1}(h/x)$$
$$\theta=\tanh^{-1}(y/x)\qquad\theta=\coth^{-1}(x/y)$$
 The number $1$ rotated one half radian in the complex plane results the imaginary unit $i$.
The number $1$ rotated one half radian in the complex plane results the imaginary unit $i$.
 The insertion of $i$ into the unit circle equation results in the unit hyperbola equation.
$$x^2+(i\cdot y)^2=1\medspace\to\medspace x^2-y^2=1$$
The insertion of $i$ into the unit circle equation results in the unit hyperbola equation.
$$x^2+(i\cdot y)^2=1\medspace\to\medspace x^2-y^2=1$$